Fill in the gaps.
6. have to
7. reached, relayed
8. site
9. He went ________ to oblige his superior. out of his way
10. put up with
Opposite word:
11. Abundant- scarce
12. Gaudy-modest
13. Accumulate- Scatter
14. Costly-economical
15. Forbid- allow
Incorrect part in the sentence:
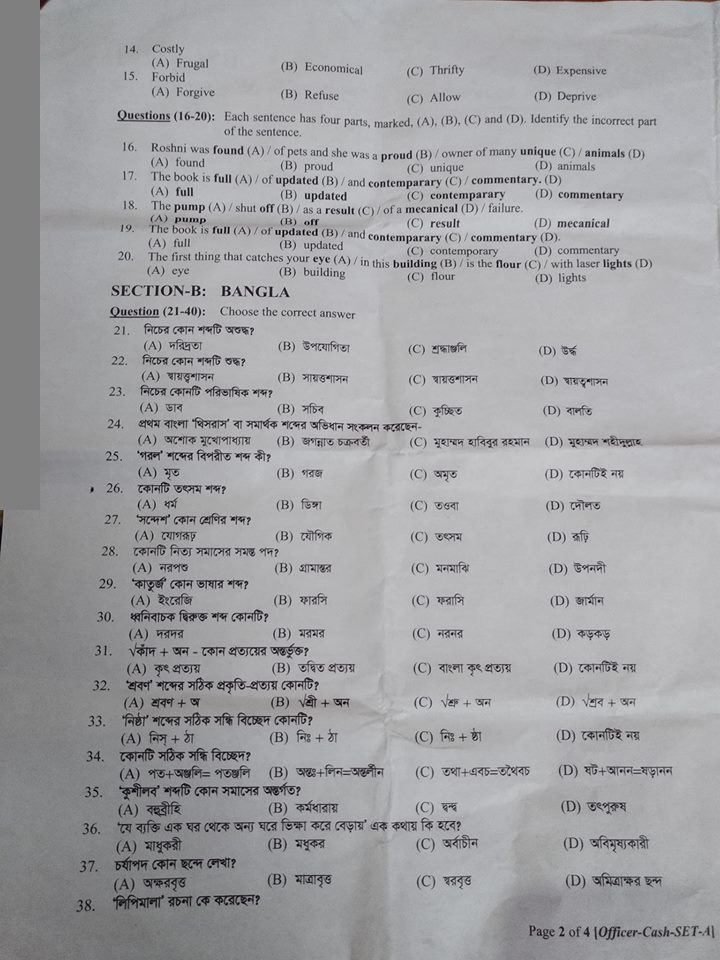
16. found
17. contemparary
18. mecanical
19. contemparary
20. flour
২১. কোনটি অশুদ্ধ- উর্দ্ধ
২২. কোনটি শুদ্ধ- স্বায়ত্তশাসন
২৩. পারিভাষিক শব্দ – সচিব
২৪. থিসরাস – অশোক মুখোপাধ্যায়
২৫. গরল এর বিপরীত – অমৃত
২৬. তৎসম – ধর্ম
২৭. সন্দেশ – রূঢ়ি
২৮. নিত্যসমাস – গ্রামান্তর
২৯. কার্তুজ – ফরাসি
৩০. ধ্বনি বাচক দিরুক্ত – কড়কড়
৩১. ♪কাঁদ +অন – কৃৎপ্রত্যয়
৩২. শ্রবন – ♪শ্রু+অন
৩৩. নিষ্ঠা – নিঃ+ঠা
৩৪. ষড়ানন – ষট + অানন
৩৫. কুশীলব – দ্বন্দ্বসমাস
৩৬. যে ব্যক্তি এক ঘর থেকে অন্য ঘরে ভিক্ষা করে বেড়াই – মাধুকরী
৩৭. চর্যাপদ – মাত্রাবৃত্ত
৩৮. লিপিমালা – রামরাম বসু
৩৯. জন্ডিস ও বেলুন – নাটক
৪০. শাহানামা – পারস্য
41. In a business A and C invested amounts in the ratio 2:1 whereas A and B invested amounts in the ratio 3:2. If their annual profit be Tk. 157300, then B’s share in the profit is:
—
A : B = 3 : 2
A : C = 2 : 1
A : B : C = 6 : 4 : 3
B’s share = 157300 × 4/(6+4+3) = 48400. Ans: D
42. The average age of a group of 15 employees is 24 years. If 5 more employees join the group, the average age increases by 2 years. Find the average age of the new employees.
—
Sum of the ages of (15+5) = 20 employees => 20×(24+2) = 520 years
Sum of the ages of 15 employees => 15×24 = 360
Sum of the ages of new 5 employees = (520-360) = 160
Average = 160/5 = 32. Ans: D
43. A person travels a certain distance at 3 km/hr and reachers 15 min late. If he travels at 4 km/hr, he reaches 15 min earlier. The distance he has to travel is:
—
D/4 – D/3 = (15+15)/60
D/12 = 1/2
D = 6 km. Ans: B
44. The difference between the radii of bigger circle and smaller circle is 14 cm and the difference between their areas is 1056 cm². Radius of the smaller circle is :
—
π(x+14)² – πx² = 1056
π(x²+28x+196-x²) = 1056
28x+196 = 1056/π = 1056×7/22 = 336
x = (336-196)28 = 5. Ans: B
45. A sum fetches a simple interest of Tk. 6000 at the rate of 5% p.a. in 6 years. What would be the compound interest earned at the same rate of interest and the same principal in 2 years?
—
The sum = Tk. (6000×100)/(5×6) = Tk. 20000
Compound Interest = (20000 × 105/100 × 105/100) – 20000 = Tk. 2050. Ans: A
46. Alam sold an item for Tk. 6384 and incurred a loss of 30%. At what price should he have sold the item to have gained a profit of 30%?
—
Required price = Tk. 6384 × (100+30)/(100-30) = Tk. 11856. Ans: B
47. Kiran purchased a scooter for Tk. 52000. He sold it at loss of 10%. With that money he purchased another scooter and sold it at profit of 20%. What is his overall loss/profit?
—
Selling price of the first scooter = Tk. 52000 × (100-10)/100 = Tk. 46800.
Loss on the first scooter = Tk. 52000×10% = Tk. 5200.
Profit on the second scooter = Tk. 46800×20% = Tk. 9360.
Net profit = Tk. (9360-5200) = Tk. 4160. Ans: D
48. A square is inscribed in a circle of diameter 2a and another square is circumscribing circle. The difference between the areas of outer and inner squares is:
—
Length of a side of the outer square = 2a.
Area = (2a)² = 4a².
Length of the diagonal of the inner square = 2a.
Length of a side of the inner square = 2a/√2
Area = (2a/√2)² = 4a²/2 = 2a²
Difference = 4a²-2a² = 2a². Ans: B
49. The average of nine numbers is 50. The average of the first five numbers is 54 and thatof the last three numbers is 52. Then the 6th number is:
—
The 6th number is = (50×9) – (5×54 + 3×52) = 450 – 426 = 24. Ans: D
50. A batsman has a certain average of runs for 12 innings. In the 13th inning, he scores 96 runs threby increasing his average by 5 runs. What is his average after the 13 innings?
—
Average of 12 innings = x
According to the question:
(12x+96)/13 = x+5
x = 96-65 = 31.
Average in 13 innings = 31+5 = 36 Ans: A
51. A man takes 3 hours 45 minutes to row a boat 22.5 km downstream of a river and 2 hours 30 minutes to cover a distance of 10 km upstream. Find hte speed of the river current in km/hr.
—
Speed of the boat = x kmph, the river current = y kmph.
3H45M = (3+ 45/60) hr = 15/4 hr
2H30M = (2+ 30/60) hr = 5/2 hr.
According to the question:
x+y = 22.5/(15/4) = 6
x-y = 10/(5/2) = 4
2x = 2
x = 1. Ans: A
52. A man can row 6 km/hr in still water. If the speed of the current is 2 km/hr, it takes 3 hrs more in upstream than in the downstream for the same distance. The distance is:
—
D/(6-2) – D/(6+2) = 3
D = 24. Ans: B
53. A train 150 m long passes a pole in 15 seconds and crosses another train of the same length travelling in opposite direction in 8 seconds. The speed of the second train in (km/h) is:
—
Speed of the first train = 150/15 = 10 mps.
speed of the second train = x mps.
According to the question:
x+10 = (150+150)/8
x = 27.5 mps = 27.5 × 3600/1000 = 99 kmph. Ans: D
54. The ratio of the speeds of a train and a man is 6:1. The length of the train is 650 m and crosses a pole in 1 minute 5 seconds. In how much time will hte man cross 240m long platform?
—
Speed of the train = 650/65 = 10 mps.
Speed of the man = 10/6 = 5/3 mps.
Time for the man to cross the platform = 240/(5/3) = 144 seconds = 2 minutes 24 seconds. Ans: D
55. If the numerator of a fraction is increased by 150% and the denominator of a fraction is increased by 200% fraction becomes 10/19. Find the fraction.
—
(x + 150% of x)/(y + 200% of y) = 10/19
2.5x/3y = 10/19
x/y = 10×3/19×2.5 = 12/19. Ans: C
56. A number when divided by 627 leaves a remainder 43. By dividing the same number by 19, the remainder will be :
—
627 is a multiple of 19. So, if a number divided by 627 leaves a remainder 43, then the same number divided by 19 will leave the same remainder as 43 divided by 19 leaves.
43 = 19×2 + 5.
So, the remainder will be 5. Ans: D
57. A can finish a work in 5 days and B takes 4 days to do the same work. If the work is increased by 8 times, how many days will it take for both of them to finish the work if they work together?
new work size = 1+8 = 9 works.
A & B can do in 1 day (working together) = 1/5 + 1/4 = 9/20
Required number of days = 9/(9/20) = 20 days. Ans: C
58. A computer can perform 30 identical tasks in 6 hour. At that rate, what is the minimum number of computers that should be assigned to complete 80 of the tasks within 3 hours?
—
Number of computers = (80×6/30×3) = 5.33 = minimum 6 . Ans: C
59. A and B together can complete a piece of work in 12 days, B and C can do it in 20 days and C and A can do it in 15 days. A, B and C together can complete it in:
—
A & B can do in 1 day = 1/12 work
B & C can do in 1 day = 1/20 work
C & A can do in 1 day = 1/15 work
2(A&B&C) can do in 1 day = 1/12 + 1/20 + 1/15 = 12/60 = 1/5 work.
A&B&C can do in 1 day = 1/(5×2) = 1/10 work.
Required number of days = 1/(1/10) = 10 days. Ans: D
60. There are 15 boys and 10 girls in a class. If three students are selected at random, what is the probability that 1 girl and 2 boys are selected?
—
1 girl & 2 boys can be selected in = (10C1)×(15C2) ways = 10×105 ways = 1050 ways.
3 students can be selected from 25 students = 25C3 = 2300 ways
Required probability = 1050/2300 = 21/46. Ans: C
61. UAE
62. United States
63. Aretha Franklin
64. China
65. Relative Humidity
66. Bilateral Trade
67. Angola
68. CFC
69. TIRPS
70. 1783
71. which of the following functions is not performed by Server? Word processing.
72. A device that connects to a network without the use of cables is said to be? None
73. Blinking point which shows the position in text is called- Cursor
74. In excel charts are created using which option- Chart Wizard
75. Which of the following is secondary memory device- RAM
76. By pressing which key you can move to the beginning of a page- Home Key
77. Which of the following is image name extension- gif
78. Typeface option will come under which menu? Format
79. Program designed to perform specific tasks is known as- application software
80. Pressing f8 key for three times selects- a sentence